Stéphane Legendre    CNRS | ENS

>Stéphane Legendre

stephane legendre
CNRS - UMR 8197
Ecole Normale Supérieure
46 rue d'Ulm
75230 Paris Cedex 05
France
Tel: +33.1.44.32.37.01
legendre@ens.fr

Research team

Team of Mathematical Eco-Evolution

Research interests

My work is at the interface of mathematics, computer science and ecology, i.e., ecological modelling.


Population dynamics

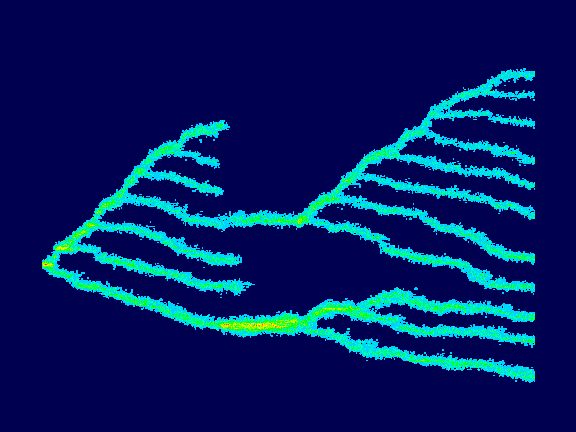
Development of the ULM computer program (Legendre & Clobert 1995, Ferrière & al 1996; Fig.1), widely used by ecologists. 

  • The ULM program can be downloaded there.

Figure1

Figure 1. Chaotic attractor in population dynamics studied with the ULM software (model from Ferrière 1992).


Conservation biology

Small populations dynamics: 

  • reintroductions (Sarrazin & Legendre 2000)
  • extinctions (Legendre & al. 1999, Schoener & al 2003, Legendre & al 2008)

Figure2

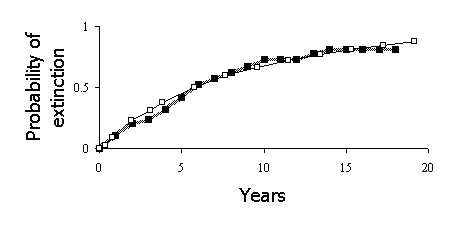
Figure 2. Probability of extinction as a function of time for the spider Argiope argentata in the Bahamas: observed (initial population size = 21-50 individuals; solid squares), and simulated (initial population size = 32 individuals; open squares). ULM model incorporating demographic stochasticity, population ceiling and environmental stochasticity (Schoener & al. 2003).


Sexual selection

Impact of sexual selection on small populations: 

  • 2-sex demographic models (Legendre & al 1999, Legendre 2004, Bessa-Gomes & al 2004, 2010),
  • The pair formation process (Bessa-Gomes & al 2003)
  • Combinatorics (Møller & Legendre 2001; Fig. 3)
  • Adaptive dynamics (Morlon & Legendre 2002)

Figure3

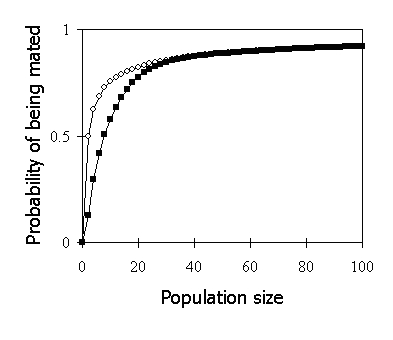
Figure 3. The probability of one individual being mated as a function of population size with monogamous pair formation, sex ratio 0.5, and chance realization in the number of males and females (circles). When each individual chooses its mate with probability 0.5, the probability of being mated is reduced at low population sizes (squares), as observed in captive populations (Møller & Legendre 2001).


Evolutionary dynamics

The ZEN computer program (Fig. 4) allows to study ecological models in an evolutionary perspective. The project was funded by an Action Incitative Bioinformatique from the CNRS. 

  • The ZEN program can be downloaded  there


Figure4

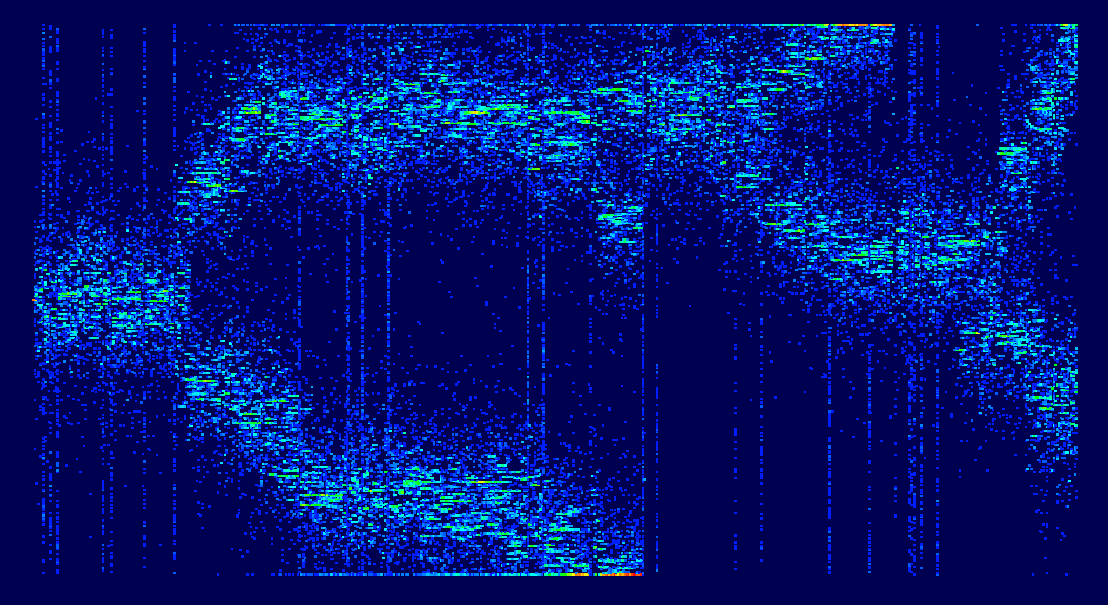
Figure 4. Adaptive radiation in bacteria with environmental catastrophes (mixing). Time on the horizontal axis, position on the vertical axis. The figure shows the positions of diversifying phenotypes adapted to different niches along a vertical gradient  (ZEN simulation; collaboration with Andy Gonzalez, Régis Ferrière and Nirmala Massin).

Figure5

Figure 5. Evolutionary restoration after environmental change. Distribution of phenotypes along time in polymorphic populations. The environment is constant over the first 21000 generations and changes gradually between time 21000 and 22000. Only 2 branches persist. The environment is progessively restored to its initial state from time 31000 to 32000. Diversity recovers. Model of cooperative evolution (Ferriere & Legendre 2013).

Allometry

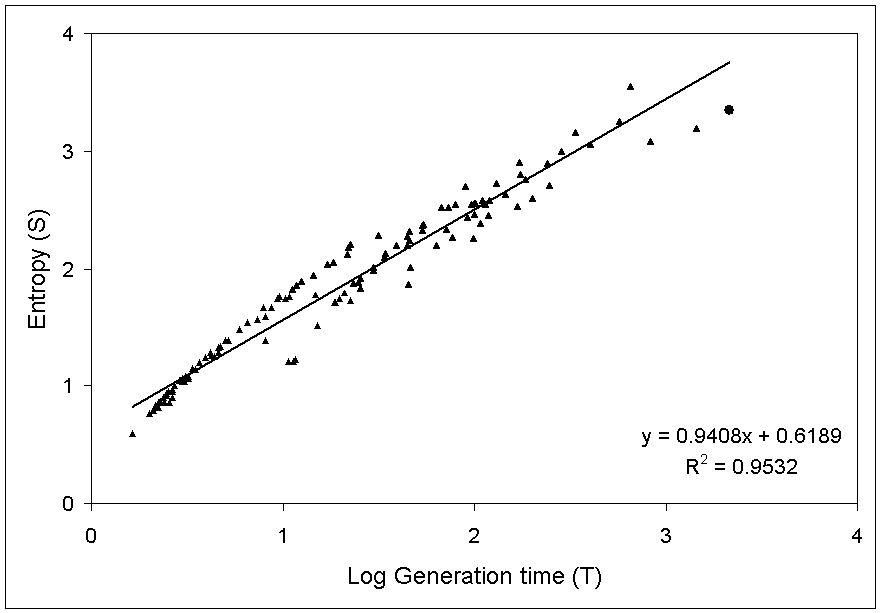
 Evolutionary entropy is a measure of the life-cycle complexity introduced by L. Demetrius. We show that entropy is in logarithmic relation with generation time (Fig. 6), and with body size. When entropy is considered as a measure of fitness, this implies that along evolutionary time, body size should increase in equilibirum species (Cope's rule), and decrease in opportunistic species (Demetrius, Legendre & Harremöes 2009).

Figure6

Figure 6. The relation S ~ log T + b for 127 species of mammals, with S evolutionary entropy and T generation time (Demetrius & al. 2009).  The large dot corresponds to Homo sapiens.

Ecological networks

Development of computer programs to study ecological networks using the methods of graph theory.

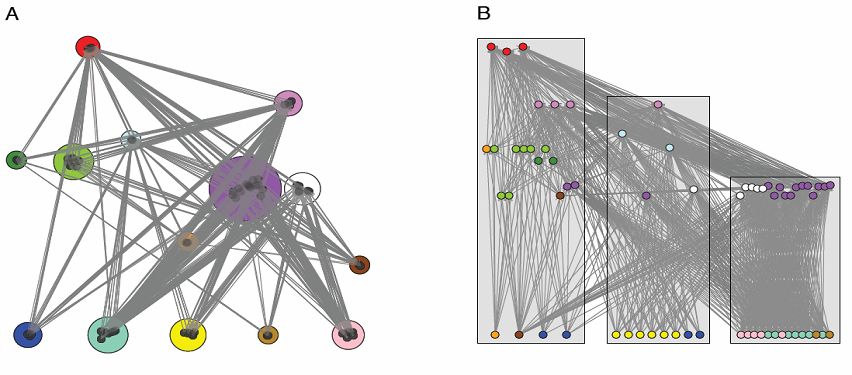
Trophic networks (food webs; Lazzaro & al 2009, Gauzens & al 2013, Gauzens & al 2015)

The n_w program  (Fig. 7), can be downloaded there. 


Figure7

Figure 7. Representation of the Créteil Lake food web with basal species at the bottom and consumer species arranged according to their trophic height . (A) Partition into trophic groups indicated by color circles. (B) Partition into modules indicated by rectangles. Trophic groups and modules decompositions computed by the n_w program.


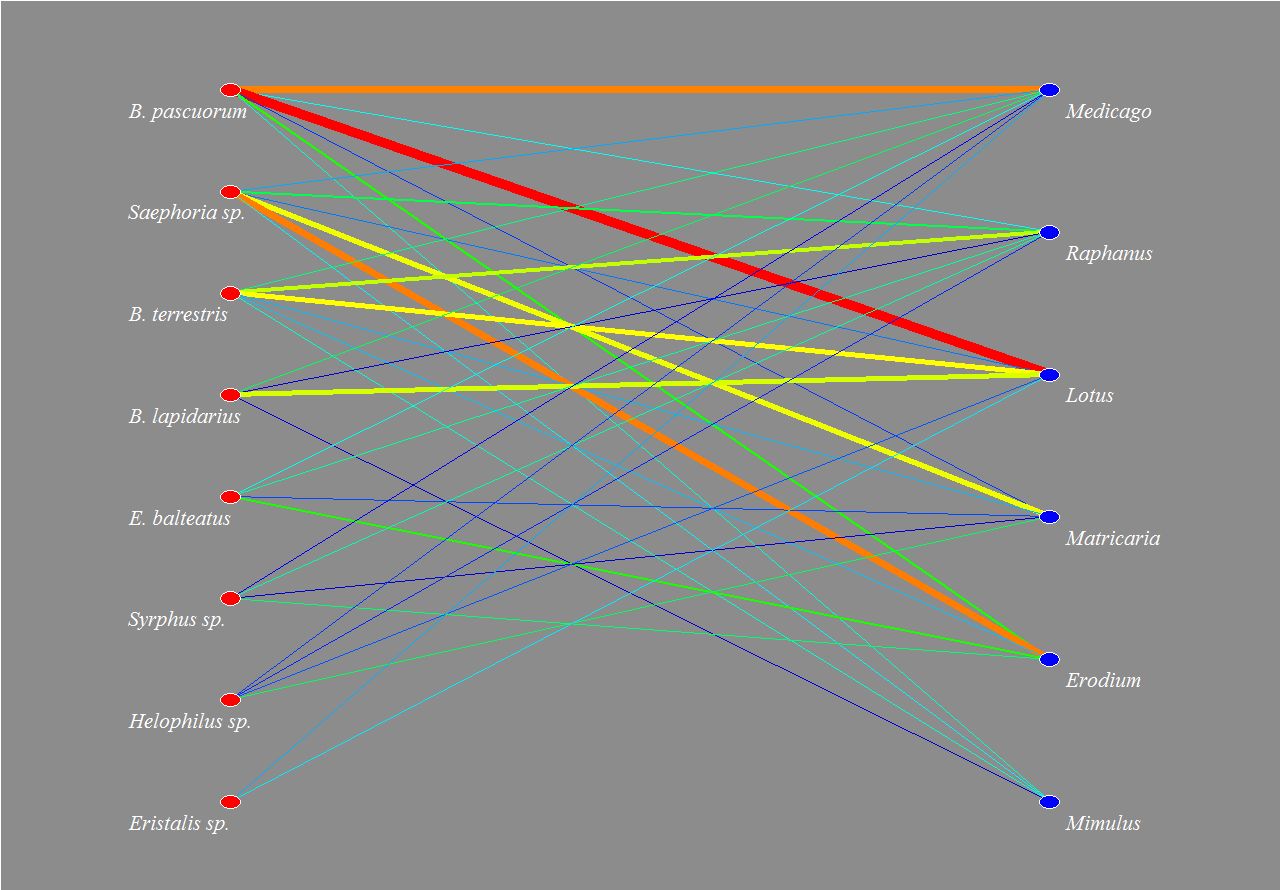
Bipartite ecological networks (plant-pollinator, site-species, host-parasite, male-female, ...)

The a2b program  can be downloaded there. 


Figure8

Figure 8. Representation of an experimental plant-pollinator network from Colin Fontaine using the a2b program. The width and color of the links correspond to the number of visits of plant species on the left by pollinator species on the right.

Publications

  • Legendre S & J Clobert. 1995. ULM, a software for conservation and evolutionary biologists. Journal of Applied Statistics 22:817–834.
  • Thomas-Orillard M & S Legendre. 1996. Virus C de la Drosophile et dynamique d'une population hôte. Comptes Rendus de l'Académie des Sciences de Paris 319:615–621. PDF file
  • Ferrière R, F Sarrazin, S Legendre & J-P Baron. 1996. Matrix population models applied to viability analysis and conservation: Theory and practice with ULM software. Acta Oecologica 17:629–656.
  • Legendre S, J Clobert, AP Møller & G Sorci. 1999. Demographic stochasticity and social mating system in the process of extinction of small populations: The case of passerines introduced to New Zealand. American Naturalist 153:449–463. PDF file
  • Sarrazin F & S Legendre. 2000. Demographic approach to releasing adults versus young in reintroductions. Conservation Biology 14:488-500. PDF file
  • Barot S, J Gignoux, R Vuattoux & S Legendre. 2000. Demography of a savanna palm tree in Ivory Coast (Lamto): population persistence and life-history. Journal of Tropical Ecology 16:637–655. PDF file
  • Møller AP & S Legendre. 2001. Allee effect, sexual selection and demographic stochasticity. Oikos 92:27–34. PDF file
  • Barot S, J Gignoux & S Legendre. 2002. Stage-classified matrix models and age estimations. Oikos 96:56–61. PDF file
  • Morlon H & S Legendre. 2002. A model of sexual selection using adaptive dynamics: The Fisher's runaway and its reversibility. Unpublished manuscript. PDF file
  • Schoener TW, J Clobert, S Legendre & DA Spiller. 2003. Life-history models of extinction: A test with island spiders. American Naturalist 162:558–573. PDF file
  • Bessa-Gomes C, S Legendre, J Clobert & AP Møller. 2003. Modeling mating patterns given mutual mate choice: The importance of individual mating preferences and mating system. Journal of Biological Systems 11(3):205–219. PDF file
  • Bessa-Gomes C, M Danek-Gontard, P Cassey, AP Møller, S Legendre & J Clobert. 2003. Mating behaviour influences extinction risk: insights from demographic modelling and comparative analysis of avian extinction risk. Annales Zoologici Fennici 40(2):231–245. PDF file
  • Chapron G, P-Y Quenette, S Legendre & J Clobert. 2003. Evaluating conservation strategies for the French Pyrenean brown bear (Ursus arctos) population by using stage-structured population models. Comptes Rendus Biologies 326:S174–S182. PDF file
  • Chapron G; S Legendre, R Ferrière, J Clobert & RG Haight. 2003. Conservation and control strategies for the wolf (Canis lupus) in western Europe based on demographic models. Comptes Rendus Biologies 326:575–587. PDF file
  • Robert A, F Sarrazin, D Couvet & S Legendre. 2004. Releasing adults versus young in reintroductions: Interactions between demography and genetics. Conservation Biology 18:1–10. PDF file
  • Bessa-Gomes C, S Legendre & J Clobert. 2004. Allee effects, mating systems and the extinction risk in populations with two sexes. Ecology Letters 7:802–812. PDF file
  • Legendre S. 2004. Influence of age structure and mating system on population viability. In Evolutionary Conservation Biology (Ferrière R, U Dieckmann & D Couvet eds.), Cambridge University Press, pp. 41–58. PDF file
  • Chapron G, DG Miquelle, JG Goodrich, A Lambert, S Legendre & J Clobert. 2005. The effect of poaching on tigers in the russian far east. In Tigers of Sikhote-Alin Zapovednik: ecology and conservation, DG Miquelle, JG Goodrich & EN Smirnov (eds.), pp. 191–195, chapter 25 (in Russian). PDF file
  • Legendre S. 2008. La séparation en biologie. In De la Séparation, directed by C Schaeffer, L'Harmattan, Paris, pp. 55–71. PDF file
  • Legendre S, TW Schoener, J Clobert & DA Spiller. 2008. How is extinction risk related to population-size variability over time? A family of models for species with repeated extinction and immigration. American Naturalist 172:282–298.
  • Chapron G, DG Miquelle, A Lambert, JM Goodrich, S Legendre & J Clobert. 2008. The impact on tigers of poaching versus prey depletion. Journal of Applied Ecology 45:1667–1674.

  • Demetrius L, S Legendre & P Harremöes. 2009. Evolutionary entropy: A predictor of body size, metabolic rate and maximal life span. Bulletin of Mathematical Biology 71:800–818. PDF file
  • Lazzaro X, G Lacroix, B Gauzens, J Gignoux & S Legendre. 2009. Predator foraging behaviour drives food-web topological structure. Journal of Animal Ecology 78:1307–1317. PDF file
  • Legendre S. 2009. The number of crossings in a regular drawing of the complete bipartite graph. Journal of Integer Sequences, Vol. 12,  article 09.5.5
  • Bessa-Gomes C, S Legendre & J Clobert. 2010. Discrete two-sex models of population dynamics: On modelling the mating function. Acta Oecologica 36:439–445. PDF file
  • Legendre S. 2011. La résilience des écosystèmes. In De la Réparation, directed by C Schaeffer, L'Harmattan, Paris, pp. 41–51. PDF file
  • Hulot F, D Carmignac, S Legendre, C Yéprémian & C Bernard. 2012. Effects of microsystin-producing and microsystin-free strains of  Planktothrix agardhii  on long-term population dynamics of Daphnia magna. Annales de Limnologie -  International Journal of Limnology 48:337–347.  PDF file
  • Demetrius L & S Legendre. 2013. Evolutionary entropy predicts the outcome of selection: Competition for resources that vary in abundance and diversity. Theoretical Population Biology 83:39–54.  PDF file
  • Ferriere R & S Legendre. 2013. Eco-evolutionary feedbacks, adaptive dynamics and evolutionary rescue theory. Philosophical Transaction of the Royal Society B 368 20120081. PDF file
  • Chaine A, S Legendre & J Clobert. 2013. The co-evolution of multiply-informed dispersal: Information transfer across landscapes from neighbors and immigrants. PeerJ 1:e44; DOI 10.7717/peerj.44. PDF file
  • Gauzens B, S Legendre, X Lazzaro & G Lacroix. 2013. Food-web aggregation, methodological and functional issues. Oikos 122:1606–1615. PDF file
  • Mugabo M, S Perret, S Legendre & J-F Le Galliard. 2013. Density-dependent life history and the dynamics of small populations. Journal of Animal Ecology 82:1227–1239. PDF file
  • Edeline E, G Lacroix, C Delire, N Poulet & S Legendre. 2013. Ecological emergence of thermal clines in body size. Global Change Biology 19:3062–3068. PDF file. Supplementary information PDF file
  • Bienvenu F, L Demetrius & S Legendre. 2013. A general formula for the generation time. arXiv.q-bio.PE
  • Gauzens B, S Legendre, X Lazzaro & G Lacroix. 2016. Intermediate predation pressure leads to maximal complexity in food webs. Oikos 125: 595-603. PDF file 
  • Brom T, M Massot, S Legendre & D Laloi. 2016. Kin competition drives the evolution of sex-biased dispersal under monoandry and polygyny, not under monogamy. Animal Behavior 113: 157–166. PDF file 
  • Bienvenu F, E Akçay, S Legendre & D McCandlish. 2017. The genealogical decomposition of a matrix population model with application to the aggregation of stages. Theoretical Population Biology 115: 69–180. PDF file 
  • Massot M, S legendre, P Fédérici & J Clobert. 2017. Climate warming: a loss of variation in populations can accompany reproductive shifts . Ecology Letters 20:1140–1147. PDF file - Supp PDF file

maize deity